Astronomical Distances and Magnitudes
In the words of Douglas Adams, the author of The Hitch-Hiker's Guide to the Galaxy:
Space is big. You just won't believe how vastly, hugely, mind-bogglingly big it is. I mean, you may think it's a long way down the road to the chemist's, but that's just peanuts to space.
The distances involved in the universe are so vast that metres or kilometers will just not suffice. We must introduce new length scales with which can span the heavens.
The Astronomical Unit A.U.
One natural and practical unit we can devise is the distance from the Sun to the Earth. This is the A.U. or Astronomical Unit. 1 Astronomical Unit = 149 598 000 km
Light Year
Moving to larger distances even the AU becomes unweildly and so the next suitable unit is the light-year. The light-year, as its name would suggest, is the distance travelled by light in one year. All electromagnetic waves travel at a speed of x 299,792,458 ms-1 in a vacuum and with an average year being 365.25 days, one light year is 299,792,458 x 108ms-1 x (365.25 x 24 x 60 x 60) s =
9.46073 x 1015 m. or 9.46073 x 1012 km.
1 lt-yr = 63 239.6717 AU
With our new measuring sticks to hand we can give a few examples of the scale of the universe.
The distance from the Earth to the nearest star (Alpha Centauri A or B) after our Sun is 4.3 ly.
The Milky Way Galaxy is about 150,000 light-years across
The andromeda galaxy is 2.3 million light-years away.
The edge of the observable universe is 46.5 Giga light years away.
The Parsec
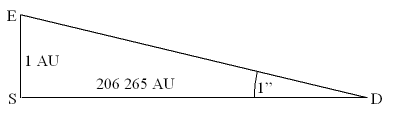
The other commonly used unit in astronomy and in Star Trek is called the Parsec (parallax of one arc second). The parsec is defined to be the distance at which a star would have a parallax angle p equal to one second of arc (1/3600 deg). The two dimensions that specify this triangle are the parallax angle (defined as 1 arcsecond) and the opposite side (defined as 1 Astronomical Unit (AU), the distance from the Earth to the Sun).
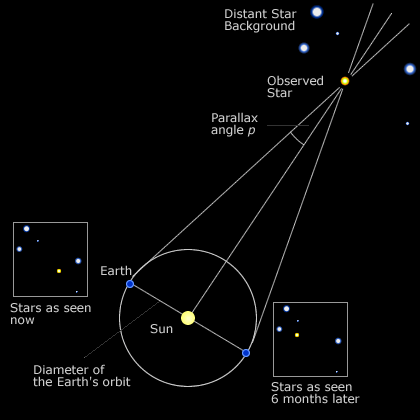
Parallax is the apparent shift in the nearest stars due to the motion of the Earth around the Sun. The method of parallax gives rise to a natural distance unit that astronomers call the parsec (which we shall abbreviate as pc).
1 Parsec = 3.08568025 × 1016 m. also used are kpc =1000 pc and Mpc =1 million pc
1 Parsec = 3.26 lt yrs.
If the star is not further than 500 light-years, then the parallax shift of the star can be used to find the distance from the Earth.
Distance (in parsecs) = 1/parallex angle.
Magnitude of Stars
Apparent Magnitude
Early Greek astronomers used a scale of magnitude devised by Hipparchus around the 2nd century BC, which was based on how bright stars appeared with the naked eye. The Hipparchus scale went from magnitude 1, for the brightest stars, up to magnitude 6, for those stars which were barely visible.
Improvements in the light gathering power of telescopes made it possible to compare the intensities of the light from stars more accurately. In 1856, Norman Robert Pogson formalized the system by defining a typical first magnitude star as a star that is 100 times as bright as a typical sixth magnitude star. Thus, a first magnitude star is about 2.512 times as bright as a second magnitude star. The fifth root of 100 (since magnitude 6 stars must be 1: x5 is known as Pogson's Ratio. In calculations, however, the factor 2.5 is often used.
To make things more confusing, the brightest stars in the sky exceed magnitude 1. These bright starts are accommodated by allowing negative magnitudes. The Sun has an apparent magnitude of -26.74, while Sirius has a magnitude of -1.46. At the other end of the scale, as the light gathering power of telescopes has increased, the magnitude scale has extended to encompass much fainter stars. The dimmest object currently observable with the largest telescopes have a magnitude of 30. As a useful reference point, the star, Vega is taken to be of 0 magnitude. More accurate measurements put its apparent magnitude at 0.03.
It is also important to note that the magnitude system is only meaningful when magnitudes are compared when measured through the same wavelength band.
| Name | Apparent Magnitude | Distance from Earth |
|---|---|---|
| Sun | -26.74 | 1 AU |
| Full Moon | -12 | 200,000 km |
| Venus | -4.71 | 38 million km |
| Sirius | -1.46 | 2.6pc |
| Vega | 0.03 | 13pc |
| Canopus | 0.7 | 96pc ±5pc |
| Faintest Stars | 30 as seen with the European Extremely Large Telescope (E-ELT) or Hubble Space Telescope | - |
The apparent magnitude m is given by
m = - 2.5 log10(b) + C(1)
Where, b is the observed intensity or brightness of the star and C is a constant, depending on the band the object is observed in, i.e. ultra-violet U, blue, B or visible V.
If we measure the brightness of two different stars, using a detector in the same band, we can determine their difference in magnitude. The difference in their apparent magnitude is given by
m1 - m2 = - 2.5 log10(b1/b2)(2)
where m1 and m2 two are apparent magnitudes of the two stars, and b1 and b2 are their respective brightness.
From the properties of logarithms, the ratio of the intensities / brightness of the two stars is.
m1 - m2 = - 2.5 [log10(b1) - log10(b2)]
m1 - m2 = - 2.5 log10(b1/b2)(3)
Absolute Magnitude
The apparent brightness of a star is how bright it seems when viewed from the Earth, but a large, bright star can appear dim if it is a long way from the Earth and a dim star can appear to be bright if it is close to the Earth. Therefore, the apparent magnitude has no bearing on the distance from the Earth.
To give an acurate measurement of the brightness of a star we need to make an absolute magnitude scale. The absolute magnitude is how bright a star is when viewed from a set distance. Stars being rather large objects, a distance of 10 parsecs was chosen.
The absolute magnitude is the brightness of a star at a distance of 10 parsecs.
Absolute Magnitude and Inverse Square Law of Intensity
In order to find the absolute magnitude, we need to know the distance of the star from the Sun. How do we do this? The intensity or brightness of light decreases with distance from the star. The rate at which it decreases is inversely proportional to the square of the distance. Thus, if we have a star of luminosity L and we move a distance d the same quantity of light has to cover a larger spherical area. Therefore, the brightness or intensity is given by
b = L/(4πd2) (4)
Or in more simple terms, the apparent brightness of the star is proportional to the 1/distance2
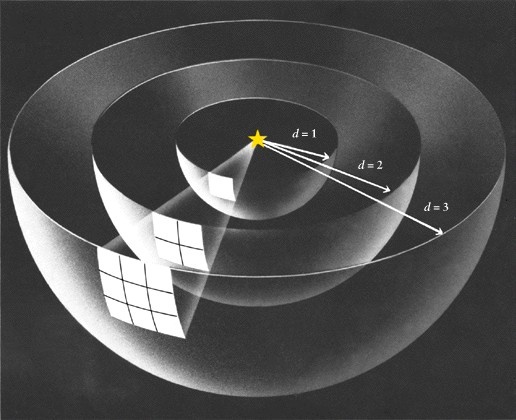
To calculate the absolute magnitude we are essentially using the relative magnitude formula and the inverse square law to allow us to substitute distance for brightness. Now we can compare its magnitude with a star at set distance of 10pc.
M- m = -2.5 log10(d2) - (-2.5 log10102)
Using the rules of logarithms to make some simplifications.
M = m -2.5 log10(d2/102)
M = m - 5 log(d/10)(5)
M = m - 5 [log(d) - 1]
M = m - 5 log(d) + 5(6)
Distance Modulus
Starting from equation (6) we can calculate the distance d from the Earth if we know the absolute magnitude. In practice we don't know the absolute magnitude because we cannot travel 10 parsecs from the star in question. We can use several indirect methods to determine its absolute magnitude. If the star is on the main sequence of stars then we can determine the brightness from its parallax.
If we know the apparent magnitude m and the absolute magnitude then we can find the distance in parsecs to the star.
m - M = 5 log10(d) + 5
Rearranging for d
d = 10((m-M)+5)/5(7)
