
The Greeks were the first to speculate that matter was discrete, in the form of particles. The word atom derives from the Greek (ατομοζ) for indivisible. Democretus, argued that matter on the large scale is composed of atoms and that different substances were composed of different atoms or combinations of atoms. Furthermore, one could substance could be converted into another simply be re-arranging the atoms. The atomic theory was roundly rejected by Aristotle, and, thus, by almost everybody else for the next two millennia.
The modern definition of an element was made in 1661 by Robert Boyle. An element is a substance that can not be broken down into simpler substances but can form compounds with other elements. There are 88 naturally occurring elements (not the much reported 92 natural elements - The elements Tc, Pm, At and Fr have no stable isotopes, and none of long half-life, so they are not naturally present.) Including man-made elements, at the time of writting (Dec, 2006) there are 117 elements. The existance of these more massive elements is fleeting with elements lasting from a few microseconds to about 30 seconds.
For the Greeks, atoms were as far as the indivisibility of matter went. However, in 1906 J. J. Thompson discovered a negatively charged particle which eventually became known as an electron. Early models the atom considered 'atom as a nice hard fellows, red or gray in color, according to taste', in which the charged particles were distributed much like the plums in a Plum pudding. However, this model of the atoms was shown to be wrong by Rutherford's experiment, in which a high energy beam of alpha particles was fired at a very thin gold foil.

If the plum pudding model of the atom was correct then the alpha particles would pass through the foil with little deflection. As shown in the figure.
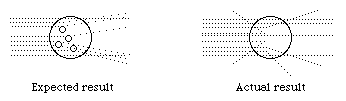
Most of the alpha particle passed through the foil with very little deflection. However, about 1 in every 8000 was scattered through an angle of more than 90 degrees. To Rutherford this was incredible. “It was quite the most incredible event that has ever happened to me in my life. It was almost as incredible as if you had fired a 15-inch shell at a piece of tissue-paper and it came back and hit you.” For the alpha particles to be scattered through such large angles and even coming back on themselves, they had to encounter a massive concentration of charged particles of very small size. The back scattering of alpha particles showed that most of the mass of the atom was concentrated at the nucleus.
The size of an atomic radius is difficult to guage since the orbits of the electrons are not in general, circular and the electron cannot be said to orbit but exists as a cloud of probability. However, taking the average distance from the nucleus as a measure of 'radius'. With the exception of the lightest elements, most atoms have radii of the order of 10-11 m. As for the nucleus, it is some 10,000 times smaller at 10-15 m. If an atom had the same diameter as the length of a football pitch (120m) then the nucleus would be about the size of a marble (1.2cm). The structure of matter is mostly empty space.
One problem with the classical theory of the atom was that with the electrons travelling around a positively charged nucleus, the electrons would be accelerating. Accelerating charges produce radiation. This radiation takes energy from the electron orbiting the nucleus and therefore the electron would spiral into the nucleus bringing about the end of atoms and the end of the universe.
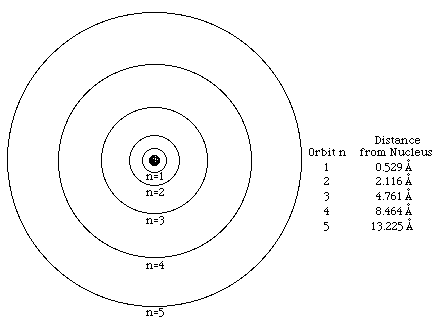
Neils Bohr considered the Hydrogen atom, as it is the simplest atom, having one electron oribiting the nucleus. The Bohr model atom propossed that the electron has certain orbits, called stationary states, which are allowed without losing energy. If the electron were to lose energy by emission or gain energy by absorption it would move to another stationary state.
To overcome this problem, Niels Bohr proposed that using Planck's quantum theory, the electron could only lose energy in fixed quantities, or quanta. The electron could only lose energy if it was one of the fixed quantities allowed by the quantum mechanics.
Bohr used his theory to explain emission spectra of Hydrogen. When heated, object emit light which when diffracted into a prism the light would be separated into discrette lines which were characteristic to each type of element. He realised that the frequency of the lines was the result of the discrette changes in energy as electron changed from one stationary state to another, in the process radiating a small quantity of energy.
| Particle | Location | Charge | Mass |
|---|---|---|---|
| Neutron | Nucleus | None | 1.008665 amu |
| Proton | Nucleus | +1 | 1.007277 amu |
| Electron | Shells around the nucleus | -1 | 0.0005486 amu |
Now we move on to a few definitions that are used in Nuclear physics. Nuclide - a combination of protons and netrons. Not all nuclides are possible but about 2500 unique combinations have been identified

In this graphic, X is a generic chemical element symbol. A represents the sum of the protons plus the neutrons making up the nucleus. It is called the nucleon number or mass number. The lower number, Z is known as the atomic number and is the number of protons in the nucleus.
The number of protons determines what the element the atom is. However, it is possible for an element to exist in more than one form by having greater or fewer neutrons in the nucleus. The different forms of the same element are known as isotopes of an element. Most elements have a few stable isotopes and a few unstable isotopes. For example: Carbon exists in 15 isotopes with the most common forms being the stable C-12,C-13 and the unstable or radioactive C-14.
There are many techniques for investigating the structure of materials. The basic concept is to direct a beam of particles at a sample and the structure can be determined by the pattern of diffraction. The physical properties of the particle beam such as charge, mass, determine what properties of the sample can be investigated.
To probe the internal arrangement of the atoms in a matterial we can use electromagnetic radiation but the wavelength must be of the same order as the spacing between planes in the material. X-rays have a wavelength that is similar to the spacing in the crystal planes.
To determine the structure of a crystal, a single crystal or powdered material is placed as a target for a columated beam of X-rays. The X-rays penetrate the crystal and some of the energy is reflected back from the different planes. The spacing of the atoms causes the X-rays to diffract. In some regions the X-rays will interfere constuctively and the combined amplitude will be must greater. In other regions, the X-rays will interfere in a destructive way and the sum of their amplitudes will be zero. Using a suitable means such as a photographic film the strength of the diffracted and reflected X-rays will shown and it is possible to calculate the structure of the material.
For amorphous substances, the arrangement of the atoms does not possess a long range order of symmetry, the X-ray diffraction pattern will be diffuse.
In crystals, the ordered position of individual atoms in a molecule will lead to a rather mysterious pattern of dark spots from which the structure can be calculated.
Quasi-crystals - materials which show some symmetry over relatively short distances, X-ray diffraction shows patterns of spots with impossible rotational orders of symmetry such as 5, 7, 13, etc.
A similar technique is that of Neutron Scattering. Neutrons carry no charge but they also have a wavelength. It might seem strange that a particle can also have a wavelength. However, this is one of the percularities of the quantum world. This idea was postulated by Prince Louis De-Broglie. Light which was thought to behave like a wave can act like a particle, maybe particles can act like waves sometimes and this is indeed the case. When the size of the particle is small then wave effects begin to become apparent. By small in this case we mean nanometer scale.
The wavelength λ of a particle is given by: λ= h/p where h is Planck's constant ~ 6.6x10-33 and p is the momentum of the particle.
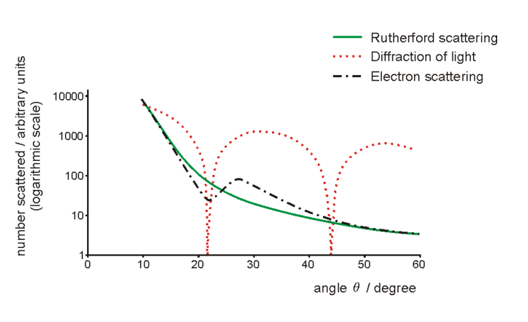
The nuclear diameter d can be calculated from the electron scattering data.
Electron beam energy = 100 MeV = 100 106=1.6x10-19 J = 1.6 x 10-11 J
Momentum p = E/c = 1.6 x 10-11/3.0 x108 = 5.3 x 10-20 kg m s-1
De Broglie relationship λ = h/p = 6.6 x 10-34/5.3 x 10-20 = 1.2 x 10-14 m
The first diffraction minimum occurs at about 22°, so using the single slit diffraction equation sinθ = λ/d we have d = λ/sin θ = 1.2 x 10-14 m /sin (22°) = 3.3 x 10-14 m
Electron beams can provide information about the surface of matterials. RHEED - Reflection High-Energy Electron Diffraction is a common tool to study the surface of growing semiconductor crystals. A high-energy electron beam is directed toward the semiconductors material. The electrons cannot penetrate the surface of the sample because of the electromagnetic force. The electrons are diffracted and form a pattern of bright and dark spots on a phorescent screen. This information can tell you about the surface quality of the material.